scienciadotcom

Acceleration

Acceleration is the rate of change of velocity. At any point on a trajectory, the magnitude of the acceleration is given by the rate of change of velocity in both magnitude and direction at that point. The true acceleration at time t is found in the limit as time interval Δt → 0.

Components of acceleration for a planar curved motion. The tangential component a_t is due to the change in speed of traversal, and points along the curve in the direction of the velocity vector. The centripetal component a_c is due to the change in direction of the velocity vector and is normal to the trajectory, pointing toward the center of curvature of the path.

In physics, and more particularly kinematics, acceleration is the change in velocity over time. Because velocity is a vector, it can change in two ways: a change in magnitude and/or a change in direction. In one dimension, acceleration is the rate at which something speeds up or slows down. However, more generally, acceleration is a vector quantity expressing the change with time of the velocity both in magnitude and in direction. Acceleration has the dimensions L T⁻². In SI units, acceleration is measured in meters per second squared (m/s²).

In common speech, the term acceleration commonly is used for an increase in speed (the magnitude of velocity); a decrease in speed is called deceleration. In physics, a change in the direction of velocity also is an acceleration: for motion on a planar surface, the change in direction of velocity results in centripetal acceleration; whereas the rate of change of speed is a tangential acceleration.

In classical mechanics, the acceleration of a body is proportional to the resultant (total) force acting on it (Newton's second law):

where F is the resultant force acting on the body, m is the mass of the body, and a is its acceleration.

Tangential and centripetal acceleration

The velocity of a particle moving on a curved path can be written as:

with v (t) the speed of travel along the path, and

a unit vector tangent to the path pointing in the direction of motion at the chosen moment in time. Taking into account both the changing speed v(t) and the changing direction of u_t, the acceleration of a particle moving on a curved path on a planar surface can be written using the chain rule of differentiation as:

where u_n is the unit (outward) normal vector to the particle's trajectory, and R is its instantaneous radius of curvature based upon the osculating circle at time t. These components are called the tangential acceleration and the radial acceleration, respectively. The negative of the radial acceleration is the centripetal acceleration, which points inward, toward the center of curvature.

Extension of this approach to three-dimensional space curves that cannot be contained on a planar surface leads to the Frenet-Serret formulas.^[1][2]

Relation to relativity

After completing his theory of special relativity, Albert Einstein realized that forces felt by objects undergoing constant proper acceleration are indistinguishable from those in a gravitational field. This was the basis for his development of general relativity, a relativistic theory of gravity. This is also the basis for the popular twin paradox, which asks why one twin ages less when moving away from his sibling at near light-speed and then returning, since the non-aging twin can say that it is the other twin that was moving. General relativity solved the "why does only one object feel accelerated?" problem which had plagued philosophers and scientists since Newton's time (and caused Newton to endorse absolute space). In special relativity, only inertial frames of reference (non-accelerated frames) can be used and are equivalent; general relativity considers all frames, even accelerated ones, to be equivalent. (The path from these considerations to the full theory of general relativity is traced in the introduction to general relativity

Illustration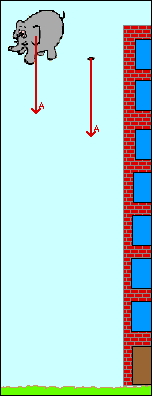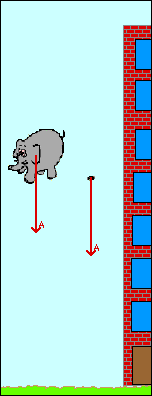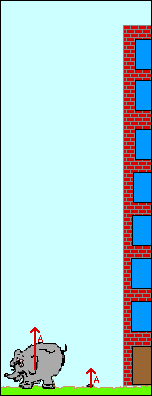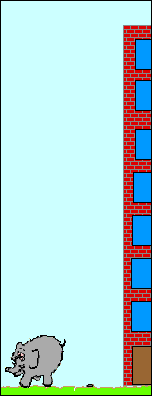

Suppose that an elephant and a feather are dropped off a very tall building from the same height at the same time. Suppose also that air resistance could somehow be eliminated such that neither the elephant nor the feather would experience any air drag during the course of their fall. Which object - the elephant or the feather - will hit the ground first? The animation at the right accurately depicts this situation. The motion of the elephant and the feather in the absence of air resistance is shown. Further, the acceleration of each object is represented by a vector arrow.

Many people are surprised by the fact that in the absence of air resistance, the elephant and the feather strike the ground at the same time. Why is this so? This question is the source of much confusion (as well as a variety of misconceptions). Test your understanding by making an effort to identify the following statements as being either true or false'

TRUE or FALSE:

The elephant and the feather each have the same force of gravity.
The elephant has more mass, yet both elephant and feather experience the same force of gravity.
The elephant experiences a greater force of gravity, yet both the elephant and the feather have the same mass.
On earth, all objects (whether an elephant or a feather) have the same force of gravity.
The elephant weighs more than the feather, yet they each have the same mass.
The elephant clearly has more mass than the feather, yet they each weigh the same.
The elephant clearly has more mass than the feather, yet the amount of gravity (force) is the same for each.
The elephant has the greatest acceleration, yet the amount of gravity is the same for each.

If you answered TRUE to any of the above, then perhaps you have some level of confusion concerning either the concepts or the words force, weight, gravity, mass, and acceleration. In the absence of air resistance, both the elephant and the feather are in a state of free-fall. That is to say, the only force acting upon the two objects is the force of gravity. This force of gravity is what causes both the elephant and the feather to accelerate downwards. The force of gravity experienced by an object is dependent upon the mass of that object. Mass refers to the amount of matter in an object. Clearly, the elephant has more mass than the feather. Due to its greater mass, the elephant also experiences a greater force of gravity.

That is, the Earth is pulling downwards upon the elephant with more force than it pulls downward upon the feather. Since weight is a measure of gravity's pull upon an object, it would also be appropriate to say that the elephant weighs more than the feather. For these reasons, all of the eight statements are false; there is an erroneous part to each statement due to the confusion of weight, mass, and force of gravity.